(19) ![]()
Increasing the nozzle pressure will cause the centerline intensity to increase linearly with pressure, as well as the gas load on the vacuum system due to molecules missing the target. To reduce the gas load on the system a collimator can be added as well as pumping between nozzle and collimator (skimmer) to avoid attenuation of the molecules by the background pressure P_b in that region. With this arrangement we basically have a jet source, but due to the low nozzle pressure the source still acts like a Knudsen cell with a collimator.
Let us now increase the nozzle pressure beyond the critical value given by equation (94) to obtain a supersonic jet. In this case the centerline intensity will be given by equation (13), repeated here:
(20) ![]()
As we can see, the gain in centerline intensity due to the "jetting action" is given by the factor:
(21) ![]()
Thus the maximum gain achievable due to the jetting action is only 3.60, given by monatomic gases (gamma=5/3, see Table 14). Therefore we can conclude that the high centerline intensity realized in a free jet source is due to high nozzle, which will be achieved by all leak valves in the limiting case of high pressures, and not due to the jetting action described by the factor given in equation (21). The skimmer and pumps used in both the Knudsen cell and jet described have the same purpose, namely to reduce the gas load on the system and to reduce Beer's law attenuation of the forward directed molecules.
A supersonic jet has other advantages over a leak valve though. The velocity of the molecules in the beam are greater and the velocity dispersion is reduced due to cooling in the continuum region of the jet expansion as described earlier (see also appendix). Therefore with a supersonic jet it is possible to achieve higher kinetic energies of better energy resolution than with a leak valve (effusive source). Note that the velocity from an effusive source is given by
(22) ![]()
whereas from a jet source by (see equations (2) and (3)):
(23) 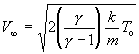
It should be emphasized again that the terminal velocity V_(infinity) does not depend on the terminal speed ration S_(infinity) or the terminal Mach number M_(infinity). It is a function of the initial gas temperature T_o and the properties of the gas used only. This fact is also explicitly mentioned by Anderson et. al. (Ref. 19). It should also be emphasized that the energy gain of jet sources is only about 2 depending on the gas specie, unless gas mixtures (seeding) is used which sacrifices beam intensity of the desired specie though. An effusive source at 3000K provides beam energies of about 0.5 eV, while a jet source at 3000K provides an energy of about 1eV.
For NH_3, the velocity from a jet is by a factor of 1.4 larger than from a similar effusive source. Also, due to the higher nozzle pressures used in a jet, molecules are much easier excited by increasing the temperature due to higher collision rates in the nozzle than in an effusive source.
Jets produce a much more monochromatic "beam" compared to effusive sources due to the cooling effect of the gas upon expansion. As can be seen from equation (6), (8), and (12) the velocity dispersion V_(parallel),_(infinity) ,_(thermal) decreases with increasing nozzle pressure P_o, nozzle temperature T_o, and nozzle diameter d.
Table of Contents